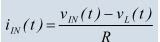Day 27 5/24/16: RMS Values, Average and Apparent Power, Power Factor, Complex Power, and Power Factor Correction

First of all, we went over what an rms value is and how to determine the rms value of different sinusoidal signals. The rms value in circuits is the equivalent dc source compared to the ac source that would provide the equivalent average power. We then did a problem of finding the rms value for a sinusoidal time-varying signal.
Next, we reviewed apparent power, average power and the power factor. Apparent power, denoted as S, is the product of the rms values of the voltage and current, or 1/2 times the maximum voltage and current. This is so because the rms values of voltage and current are max voltage/current divided by the square root of 2. Moving on, the average power is the apparent power times the power factor, or the cosine of the difference between the phase angle of voltage and current. The power factor is also the cosine of the angle of the load impedance. We then did a problem involving these different powers.
After, we learned about complex power, which is given by multiplying half of the voltage by the complex conjugate of the current. It is also given by multiplying the apparent power by the sine of the phase difference between voltage and current. The apparent power is given by the average power plus j times the complex power.
We then learned about the power triangle, which provides the apparent power (hypotenuse), the average power (leg on x-axis), complex power (leg parallel to y-axis) and the power angle. When the triangle is in the 4th quadrant, the load is capacitive and therefore the pf is leading. When the triangle is in the 1st quadrant, the load is inductive and therefore the pf is lagging. We then did a problem involving the power triangle.
Next, we learned about power factor correction in that the power factor can be increased simply by installing a capacitor in parallel to an inductive load, since purely inductive loads operate at low pf. A problem was then solved involving power factor correction. We then did a lab titled "Apparent Power and Power Factor Correction".

LECTURE:

Above is the derivation for the rms value for current. All other rms values follow the same equation, with the fact that a value other than current is replaced with the current in this equation.

In this problem, the objective was to determine the rms value for this sinusoidal wave. The formula for rms was used in this problem, with the value in the formula being the equation of this time-varying signal, or 2Vp*sin^2(wt). The rms value was found to be the square root of 1.5 times Vp.

In the above picture, we came up with an acronym to remember the phase angles of voltage and current for inductors and capacitors. This acronym is Eli the Iceman. Eli tells use that the inductor's voltage (emf) leads the current by pi/2. Ice tells us that the voltage (emf) of the capacitor lags the current by pi/2.

In this example, the apparent and average power of the load (30 ohm resistor and 0.5 H inductor) were determined, along with the power factor.

In this example, using the provided voltages and currents the apparent power, average power, complex power, power factor and impedance was determined. These values were then plotted on a power triangle. Because the power triangle is in quadrant iv instead of i, it indicates that the load is capacitive (or a leading pf).   

In this problem, the necessary capacitance was determined in order to bring the power factor from 0.8 up to 0.95. First, the difference in complex power before and after the power factor correction was determined, then it was divided by the frequency and the rms voltage squared to determine to need a capacitance of 310 uF to bring up the power factor from 0.8 to 0.95. 

LAB:

Apparent Power and Power Factor Correction:

Purpose:

The purpose of this experiment was to analyze apparent power and power factor of a load and to determine the effects of changing the resistance of the load on the apparent power, average power and power factor. The load is connected to a resistive network. Expectations were determined and those were tested on a real circuit and via measurements. It was expected that a larger difference between apparent and average power correlated to a small power factor and that the power provided to the load would be much less.
Prelab:

In the prelab, different values were determined at different resistances for the load. The inductive reactance, however, stayed the same, along with the transmission resistance. In the prelab, the RMS current delivered by the source, the RMS load voltage, the average power delivered to the load, the apparent power delivered to the load, the load's power factor, the average power dissipated in the transmission resistor and the ratio between the average power dissipated in the transmission resistor and the average power delivered to the load were determined. 

Procedure/Data:

First, the circuit seen in the prelab was first constructed, as seen above. In this picture, the 10 ohm resistor is placed in the load impedance. A wavegen channel was used to aply the input voltage. Channel 1 was used to measure the input voltage and channel 2 was used to measure the voltage across the load. The oscilloscope window was then used to determine the input voltage and voltage across the load. A math function ws also created to determine the current through the circuit. This oscilooscope window for the 10 ohm load resistor is shown below.

The same was then done with a 47 ohm load resistor instead of a 10 ohm load resistor. The reactance of the inductor stayed constant throughout the whole experiment.Below is the circuit with the 47 ohm load resistor.

Again, Ch1 was used to measure input voltage and Ch2 was used to measure the load voltage. The oscilloscope window is shown below along with a math channel to read current.

Again, the same setup and procedure was done again, with the exception that now a 100 ohm load resistor was used in replacement with the 47 ohm load resistor. The circuit is shown below.

Below is the oscilloscope window for this circuit. The same channels were used as prior.

The oscilloscope windows were then used to determine the data obtained and needed from this experiment. This data is the same as that calculated in the prelab. This data is shown in the data analysis section below.

Data Analysis / Conclusion:

Above is the data obtained from the oscilloscope windows measured in the procedure and displayed in the data section. What's inside the table is the expected results, and what's outside is the obtained data from the experiment. Looking at the 10 ohm load resistor, the experimental load voltage (840 mV) is larger than the theoretical (626 mV), with a percent difference of 34.2%. It is unknown exactly why this might have occurred, but it is possible that because small impedances were used, any small change in the resistance or reactance resulted in a larger change in voltage and current. Agsain, the current is the same; a percent difference of 36.8% between the expected (19 mA) and the experimental (26 mA). This also applies to the other trials involving the 47 ohm and 100 ohm load resistors, which helps verify that it is most likely due to the inductor not being 1 mH but actually deviating from it. Since the inductance is very small, the change affected the results dramatically.

Below is the experimental power factor, apparent power, average power, power dissipated by the transmission resistor, and the ratio between the transmission power and load power.

As can be seen, with an increasing load resistor, the power factor increases along with the ratio between transmission power and load power. However, the apparent power decreases with increasing load resistance. An interesting note is that the average power for both the load and transmission is largest when the load resistance is at 47 ohms.

5/19/16 Day 26: Op Amp AC Circuits, Oscillators, Instantaneous and Average Power and Max Power Transfer

We first quickly reviewed how to solve for op amp ac circuits, which involve nodal analysis and complex algebra. We the did a problem involving ac in op amp circuits. We then learned about oscillators, which convert dc inputs to ac outputs. This is done by using an op amp circuit with a gain of one or greater and by resulting in no phase shift between input and output. Another interesting part of oscillators is that their op amp has two feedbacks and not just one. We then reviewed a Wein-bridge oscillator, the simplest oscillator. After, we did a lab on the op amp relaxation oscillator. Lastly, we went over average and instantaneous power. Average power was found to be 1/2VIcos(v-i), where v and i are phase shifts for the voltage and current, respectively. We then did a problem involving power. Lastly, we quickly reviewed max power transfer for ac circuits, which occurs when the load impedance is the complex conjugate of the Thevenin impedance. The max power is also given by (V_th)^2/(8R_th).  

LECTURE:

In this example, the output voltage of this op amp ac circuit was determined. As in all op amp circuits, nodal analysis was used. The exception in this case is that impedances and time-varying voltages were taken into account. The answer was determined to be 1.029cos(1000t+59.04) V.

In this derivation above, the simplest Wein-bridge oscillator was solved for. It was found that for the resistance to be equal to the capacitance, the gain of such an oscillator is three and its angular velocity is 1/3. For such an oscillator, there is no imaginary part, only real values.

In this problem, the objective was to determine the average power each circuit element absorbs. Right off the bat we can tell that the average power absorbed by the capacitor and inductor are zero, since only resistors absorb an average power; capacitors and resistors absorb and release and equal amount of power, making the average zero. Then, the voltages and currents across each resistor were determined, and by this, the power was found. It was found that the source supplies 7.5 W of power, the 4 ohm resistor absorbs 5 W, and the two ohm resistor absorbs 2.5 W. This is expected, since the sum of the power in the circuit must be zero.

LAB:

Op Amp Relaxation Oscillator:

Purpose:

The purpose of this experiment was to generate a relaxation oscillator and to analyze its output behavior. A relaxation oscillator that would generate oscillations close to the expected (given from EveryCircuit) was also another goal of this lab.

Prelab:

The purpose of the prelab was to determine the resistance value of inverting feedback resistor needed to generate a frequency of 159 Hz in the relaxation oscillator. The other two parallel resistors were assumed to be equal. Beta was found to be a half and from this the resistance was determined from the period function T = 2 R C ln ((1+beta)/(1-beta)). 

Then, the determined resistance (2.86 kOhm) was tested using EveryCircuit by determining the resulting frequency of adding that value of resistance. Based on EveryCircuit, the frequency was found to be 158 Hz using such a resistance, which is very close to the needed value of 159 Hz.

Procedure:

The circuit was put to the test by first building it, as seen above. The schematic of this circuit is seen in the prelab above. For the two parallel resistors, a value of 1 kOhm was used. In addition, a 1 uF capacitor was also used. Then, using Waveforms, the output voltage and the voltage across the capacitor were measured on the oscilloscope window. The windows are shown below in the data section. Also, a 3 kOhm resistor was used instead of 2.86 kOhm, since that was the closest resistance value available.

Data:

Above is the oscilloscope window for the voltages as functions of time across the capacitor and the output voltage. The yellow graph is the voltage across the capacitor, and the blue is the output. As can be seen, the op amp goes repeatedly through positive and negative saturation, creating a square wave. In addition, comparing this window to the obtained graph in EveryCircuit, they are very similar, which shows that the procedure was performed correctly.

Data Analysis / Conclusion:

Based on this window, the frequency was experimentally calculated. The calculation is shown below: 

 As can be seen, the experimental frequency (163.7 Hz) was close to the expected (159 Hz), which shows that the method used to analyze oscillators is correct. There is a percent difference of 2.96%, and most, if not all, of this small difference is due to using a 3 kOhm resistor instead of a 2.85 kOhm.

5/17/16 Day 25: Sinusoidal Steady State Analysis in AC Circuits and Phasors: Passive RL Circuit Response

In class we learned how to apply all of the previously learned circuit analysis techniques to AC circuits instead of DC circuits. We learned how to apply nodal analysis to AC circuits, which is very similar to DC with the exception that impedance is used instead, and that everything is done in complex form, which requires complex algebra. A problem involving nodal analysis was then performed. We then learned about mesh analysis, which is exactly like mesh analysis in dc with similar exceptions as in nodal analysis. A problem involving mesh analysis in ac was then performed. After, we did a lab titled "Phasors: Passive RL Circuit Response".

Next, we reviewed superposition in ac circuits. It is very similar to superposition in dc, with the exception that impedance is used and therefore complex algebra, as in the other analysis techniques in ac. Lastly, source transformations and Thevenin and Norton equivalents were also reviewed, which involve the same concepts as in dc but the same exceptions as in the other analysis techniques in ac.

LECTURE:

In this problem, nodal analysis was used in ac circuits to find the current across the 0.1 uF capacitor. There are only two viable voltage nodes, which were used for the analysis.

In this problem, the objective was to find the current across the 4 ohm resistor. This was achieved using mesh current analysis, and again, because we are in ac, more complex algebra was seen.

In this problem, superposition principle was used to find the current across the 4 ohm resistor. As can be seen, this is the same circuit that was analyzed in the previous example. The same circuit was used to verify that superposition principle and mesh analysis can be used in ac circuits correctly. The same conditions apply for superposition in ac as in dc; current sources act as open circuits and voltage sources act as short circuits.

In this simple problem, source transformations were practiced in simple ac circuit analysis. They ae performed the same exact way as in dc circuit analysis, with the exception that now complex algebra must be used.

The Thevenin equivalent of this circuit was determined using Thevenin's Theorem and impedance equivalents. The pair of impedances in series were added to obtain equivalent impedances, and then KCL and KVL was performed to solve for the Thevenin voltage and Thevenin impedance.

LAB:

Purpose:

The purpose of this experiment was to analyze the steady-state response of an RL circuit with AC signals applied. The frequency was the same throughout the entire circuit, but each component had a different phase angle and amplitude. Because they are all at the same frequency as well, the amplitude gain between the input and output could be determined. The experimental values were confirmed by comparing to theoretical values solved for in the prelab.

Prelab:

In the prelab, the amplitude gain and phase difference were derived for an RL circuit. Then the cutoff frequency of this circuit was solved for with the given resistance and capacitance values. The cutoff frequency is R/L for an RL circuit. Next, the amplitude gain and phase difference at a tenth of the cutoff frequency, at the cutoff frequency, and at ten times the cutoff frequency were calculated. Then, the behavior of the inductor at those frequencies was predicted and used to prove the calculated amplitude gain. At low frequencies, the inductor acts close to a wire, providing a very large amplitude gain. On the other hand, at high frequencies, the inductor acts close to an open cicuit, providing a very small amplitude gain.

Apparatus:

The equipment of this experiment included: a breadboard, an inductor, a resistor, wires, an analog discovery and a laptop with Waveforms.

Procedure:

First, the RL circuit, consisting of a 47 ohm resistor in series with a 1 mH inductor, was created. Then, the wavegen on waveforms was used to apply a sinusoidal input of 2 V at the frequencies given by a  tenth of the cutoff frequency, the cutoff frequency itself, and ten times the cutoff frequency. Channel 1 was used to measure the input voltage, and channel two of the oscilloscope was used to measure the voltage across the inductor. Then, a math channel was used to determine the current through the circuit. The formula is shown below:

Below is the oscilloscope window for a tenth of the cutoff frequency:

As can be seen, the voltage across the inductor is very small, which shows that it acts close to a wire under low frequencies. The oscilloscope window for the cutoff frequency is shown below:

Lastly, the oscilloscope window for 10 times the cutoff frequency is shown below:

As can be seen, the voltage across the inductor is roughly equal to the input voltage, which shows that at high frequencies it acts like an open circuit, eating up almost all of the voltage provided.

All of the data obtained form analyzing the windows is shown in data below:

Data:

Above is the data obtained from the oscilloscope windows. Again, it is expected that the voltage drop across the inductor at low frequencies is small, because the inductor acts close to a wire. At high frequencies, it is expected that the inductor eats up nearly all of the voltage applied, since it acts as an open circuit at high frequencies. Therefore, it is also expected that the current is very small at these high frequencies.

Data Analysis/Conclusion:

Analyzing the data obtained to the calculations in the prelab, all of the obtained data is very close to the calculated values, which shows that the circuit analysis used in this experiment is correct. It is expected that the gain at low frequency is the largest and the gain at high frequency is the smallest, since at low frequencies the inductor acts as a wire and at high frequencies the inductor acts as an open circuit. In addition, the phase shifts obtained experimentally are very close to the determined values, which shows that the analysis is correct. Unfortunately, there is no conceptual way to determine the phase shift at the frequencies as there is for the gain and current.

Day 24 5/12/16: Impedance and Admittance, KCL and KVL in Frequency Domains and Equivalent Impedance

We first learned more about impedance, which is the equivalent of resistance for alternating current. We learned that impedance has both a real and imaginary part, with the real portion being the resistance of the resistor and imaginary portion being the reactance of the the capacitor and inductor. The reactance of an inductor is jwl and that of a capacitor is 1/jwC. We also learned that at very high frequencies, an inductor acts like an open circuit and a capacitor acts like a short circuit. We also learned about admittance, which is the inverse of impedance. It contains a real portion, which is the conductance, and an imaginary part, which is called susceptance. The sum of the conductance and susceptance is the inverse of the sum of resistance and reactance. We then did two problems involving finding current and voltage using impedances.

After these two problems, we did a lab titled "Impedance", which is explained in more detail in the "LAB" section below. We then reviewed Kirchhoff's laws in circuits with alternating current, which are used exactly the same as in dc current. KVL and KCL were found to hold for phasors as well.

We then learned about impedance combinations. It was found that impedances in series add, and the equivalent impedance in parallel is 1 over the sum of the inverse of the individual impedances. We then did a problem involving finding the equivalent impedance.

Lastly, the concept of phase shifters was gone over slightly. A problem was then done using phase shifters.


LECTURE:

Above is shown the magnitude of the impedance and the angle formed by the phasor as functions of resistance and reactance. This is found using polar coordinates.

The objective of the above problem is to find the current in the circuit and to find the voltage across the capacitor. First, the impedance of the circuit was found, and the current was obtained by dividing the voltage by the impedance of the circuit. The voltage of the capacitor was then obtained by multiplying the current by the impedance of the capacitor alone.

In the above problem, the objective was to find the voltage across the capacitor. This was done by multiplying the current by the impedance of the capacitor.

The objective is to find the equivalent impedance of this circuit. The impedance of the capacitor and resistor were added, and then the equivalent impednance was found by inversing the sum of the inverse of the impedance of the inductor and the previous impedance combination found.

In this problem, the voltage across the inductor was the objective to determine. First, the equivalent impedance for the inductor and capacitor was determined. Then, a voltage divider was used to find the voltage across this equivalent impedance. Because the capacitor and inductor are in parallel, it is know that the voltage across both is equal.

In this derivation, the phase shift and gain for a specific case when the resistor is equal to the reactance of a capacitor in an RC circuit was found. The phase shift was determined to be -45 degrees and the gain 0.5 of the input voltage. 

LAB:

Purpose:

The purpose of this experiment is to determine the impedance of a resistor, inductor and capacitor experimentally. Another purpose is to determine the gain of each component experimentally and the phase shifts between the current.

Prelab:

In the prelab, the theoretical current through the circuit, the voltage across the circuit elements under analysis (resistor, capacitor, inductor), the gain as a result of each element and the phase shift were determined. Above is the calculations for the resistor and the inductor. The calculations for the capacitor are shown below:

All of this data was then summarized in the table below:

As a correction, the phase shift for the inductor should be 90 degrees instead of 82.4, and the phase shift for the capacitor should be -90 degrees instead of -7.9.

Apparatus:

The equipment of this experiment consists of a breadboard, an analog discovery, resistors, a capacitor, an inductor, wires and a laptop with waveforms software.

Procedure:

First, all of the above schematics consisting of  RL, RC and resistor circuits shown above were built on one breadboard. Then, using the circuit with resistors only, a sinusoidal input with an amplitude of 2 V and an offset of 0 V was applied. This voltage input was applied at frequencies of 1 kHz, 5 kHz, and 10 kHz. The oscilloscope channel 1 was then used to measure the current through the circuit by measuring the voltage across the 47 ohm "internal" resistor then dividing that value by its resistance. The voltage across the resistor under analysis was measured using channel 2 of the oscilloscope. An image of the oscilloscope for the resistor circuit is shown below:

Using the oscilloscope window, the current through the circuit, the gain and the phase shift were determined, which is shown in the data section. The same procedure was done three times for this circuit, with each time at a different frequency.

The same procedure seen above was performed for the inductor at all three different frequencies as well. The oscilloscope for the inductor is shown below:

Again, the same procedure was done for the capacitor verbatim. The oscilloscope window for the capacitor is shown below:

Data:

Above is the data obtained from the oscilloscope window for the resistor circuit. The same data was measured at all of the three different applied frequencies of 1 kHz, 5 kHz and 10 kHz. This data includes the current function, the voltage function across the resistor under analysis, the experimental gain of the circuit and the phase shift between the voltage of the circuit element and current.

Below is the same data at the same frequencies for the inductor and capacitor, respectively..

Inductor

Capacitor

Analysis/Conclusion:

Analyzing the data of the resistor circuit, the current measured through the circuit for each trial (13.39 mA, 13.65 mA, and 13.4 mA for the 1 kHz, 5 kHz and 10 kHz) is very similar to the theoretical (13.6 mA), which shows that the analysis and set up were correct. The slight deviations are due to uncertainties in the values of the circuit elements. Because the currents are similar, the voltages are also similar. The experimental gains obtained (0.695 for 1 kHz, 5 kHz and 0.68 for the 10 kHz) are also very similar to the theoretical gain of 0.68, which is expected. Again, the deviations are due to uncertainties in the element values and error in measuring values in the oscilloscope. Lastly, the phase shift for both values is 0, which is expected for a resistor.

Analyzing the data for the inductor, the experimental current for 1 kHz (40.5 mA) is similar to the theoretical (42.2 mA), and the difference is due to human uncertainty when obtaining values from the oscilloscope and uncertainty in the values of the circuit elements. It was found that the current and voltage varies depending on the frequency, which is expected because the voltage and current are related and time-dependent by v = Ldi/dt. Because the voltage differs depending on the frequency, the gain also differs. However, for the 1 kHz, the obtained gain (0.127) is close to the theoretical gain of 0.133.  Lastly, the experimental phase shift (88.2 degrees) is also close to the theoretical (90 degrees).

Analyzing the data for the capacitor, the voltage, current and gain also differ depending on the frequency, because they are time-dependent and related by i = Cdv/dt. However, analyzing at 1 kHz frequency, the experimental current (4.7 mA) is deviating from the theoretical (5.8 mA). It is unknown exactly why, but it is thought that it could be due to a large uncertainty in the capacitance, since the experimental gain and theoretical gain turned out to be exactly the same (0.99). In addition, the experimental phase shift is also exactly equal to the theoretical (-90 degrees).

Above is the experimental and theoretical impedances for each circuit element under the different applied frequencies, along with the percent differences between these frequencies. The percent differences are large for some of the capacitor values and for the inductor values because of uncertainty in the element values. Because the elements we were working with are small, any small change results in a big difference in impedance.